#It's complicated
It's complicated
While skoormit has pointed out that the average percent increase for 1 die is 21.43, the overall increase for multiple dice is not straightforward, and highly depends on your Charisma modifier, more so for more dice.
I used a small python script to roll xd6. The lowest c dice (where c = positive Cha modifier) were re-rolled, unless there were less than c dice below average. The following data was gathered by averaging over 100,000 of such sets for each combination of x and c.
It shows the percentile increase of the average roll, i.e. ((new avg / old avg) − 1) × 100.
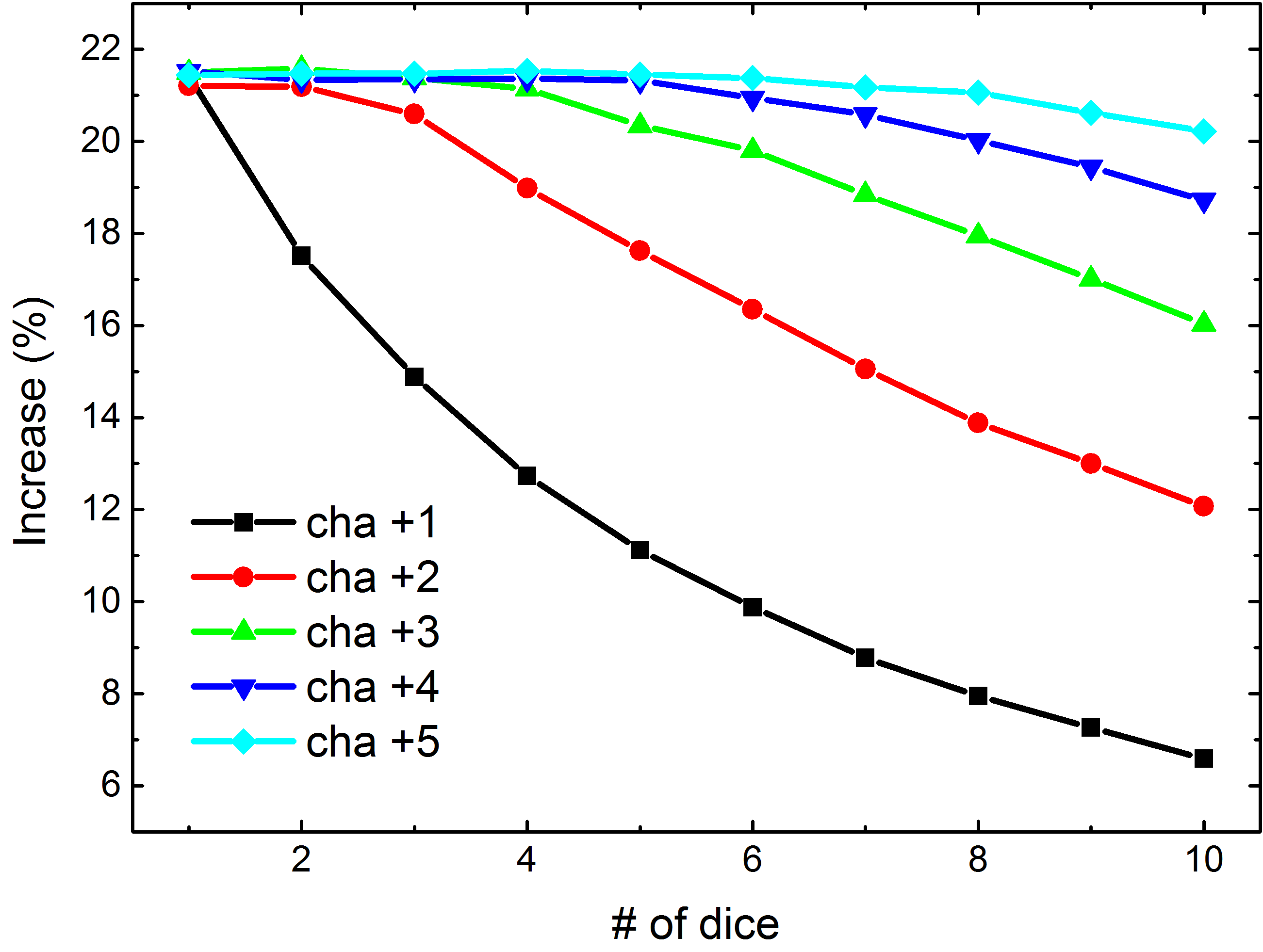
As you can see, the increase drops rapidly below 20% for x > c, though the slope becomes less steep as c increases.
