TLDR: Yes the Great Sword is superior to the Great Axe because it has more dependable damage output. So you will kill enemies with fewer hits.
Even when you boost the average damage from the great axe beyond the great sword, this is done by having a higher chance to critically hit and deal more damage on average by a critical hit. Yet was this extra damage from a critical hit actually needed to kill the enemy? Or are you using the same number or even more attacks to fish for that critical hit, if normal hits would have killed the enemy in fewer tries.
Especially as a Barbarian, Reckless Attacks does expend a resource: your health, because you will be that much easier to hit and damage. If that is your plan for being the tank, more power to you.
Introduction
In my current DnD 5e campaign I play a half-orc barbarian and I wanted him to be wielding the most iconic weapon: the great axe.
The problem explained in other responses here, quickly became apparent to me: there is no statistical advantage to wield a great axe over a great sword. In former editions the Great Axe had some appeal by having a x3 critical hit multiplier instead of the regular x2.
So I went on looking in the current rules to find some features to make 1d12 a good choice.
A principle I use to determine the probability value of a weapon is the expected range, which is the range of results where 75% of the rolls end up in. I'll come back to this when I have a graph to make this more clear. Yet for 2d6 this is meaningful, as it has a clear median (7, which is the result most rolled 1/6 times). For a 1d12 roll this is useless as all results have the same probability.
Looking at some options to mitigate the problems of 1d12 weapon
The first place I went looking was Fighting styles, I though reducing the chance of a 1-2 result would go a long way, reducing crappy rolls. Yet if we look at the graph below, were the impact of Greatweapon fighting is shown, we see that the Great Sword benefits more from this fighting style as it let's you re-roll all 1's and 2's from a roll. (IMHO the better way would be to grant one re-roll per damage roll).
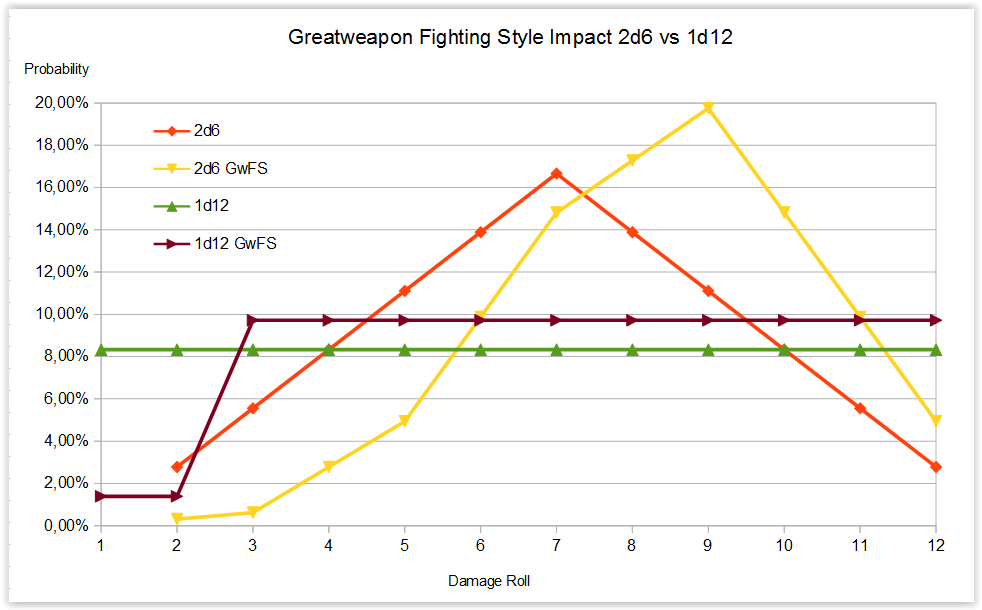 I have connected the data points with lines for a more visual representation. So when one 'curve' is above the other, those result are more likely to occur than those beneath it. This shows that on a natural roll a great axe has higher probability to roll 11-12 than 2d6, yet with GW fs this is reduced to only 12.
I have connected the data points with lines for a more visual representation. So when one 'curve' is above the other, those result are more likely to occur than those beneath it. This shows that on a natural roll a great axe has higher probability to roll 11-12 than 2d6, yet with GW fs this is reduced to only 12.
If we translate this to expected results. For 2d6 we get the following:
83,33% falls between 4-10 for a natural roll, 86,42% falls between 6-11 with GW fighting style. With the minimum and median boosted +2 and the maximum +1, this is a significant increase and the average increase of 1,33 is somewhat misleading here as the floor goes up more than the ceiling, this fighting style makes the Great Sword even more dependable with results of <=4 having only a probability of 3,7%!
The 1d12 Great Axe has the following increases:
83,33% fell between 2-11 on a natural roll, with GW fs this becomes 77,78% between 4-11 or 97,22% between 3-12. This does improve the floor by +2 but the ceiling doesn't really move. Which is confirmed by the average increase of 0,83. So this fighting style does reduce the chance of rolling <=2 to 2,78% yet the minimum isn't increased all that much. So the variability isn't reduced and thus the reliability of the Great Axe is still lacking.
Next stop: Savage Attacker feature
By now most people know this feature to be a trap feature but I still went to see what it's impact is, because a re-roll is always useful if you are looking for a minimum result.
So let's take a graphic representation of this:
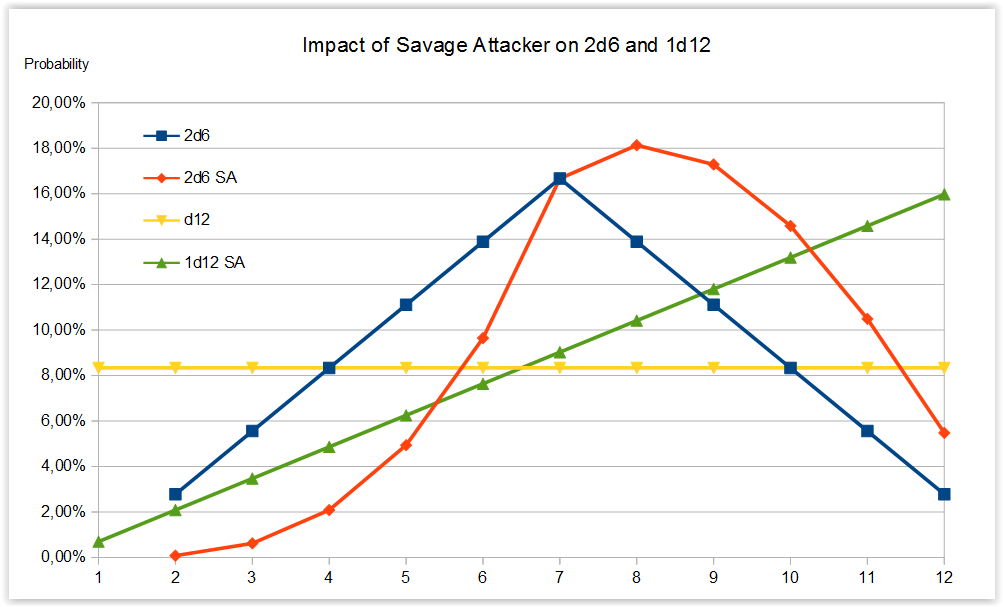 This is the chance for one attack, which is of course the weak spot of this feature: it only helps for one bad roll, if you roll twice bad for damage, you are going to be stuck with the second bad roll. Also if you don't manage to roll higher than the previous roll, there is nothing gained by this feature. This is why I came up with a homebrew version of it, which grants 3 advantages:
This is the chance for one attack, which is of course the weak spot of this feature: it only helps for one bad roll, if you roll twice bad for damage, you are going to be stuck with the second bad roll. Also if you don't manage to roll higher than the previous roll, there is nothing gained by this feature. This is why I came up with a homebrew version of it, which grants 3 advantages:
- Half ASI STR or DEX
- One reroll per round for melee weapon damage, but if the re-rolled result <= the initial result you can add half max damage die to it. So with 1d12 you add 6 and with 2d6 you add 3 to the re-rolled lower result.
- You get one critical melee weapon damage re-roll per round.
As we can see in the graph, once again 2d6 tends to gain more from a damage re-roll as the lower parts of the rolls get ignored for the more average or higher roll. 1d12 Does keep it's advantage now for rolling more times 11-12 but naturally still ends up more with low rolls <= 5. So if you would use the stock Savage Attacker feature, I would only re-roll any results <=4 on the first damage roll of the turn.
Let's have a look on the expected results for 2d6:
Note that the expected ranges are 4-10: 83,33% and 6-11: 86,61% respectively
- Minimum moves from 4 to 6: a gain of +2
- Median moves from 7 to 8: a gain of +1
- Maximum moves from 10 to 11 a gain of +1
On average 1,37 more damage but this clearly doesn't tell the whole picture as the lower results become far less frequent.
For 1d12 we see the following changes:
Note that the expected ranges are 2-11: 83,33% and 6-12: 82,64% respectively
- Minimum moves from 2 to 6: a gain of +4!
- Median moves from 7 to 12: a gain of +5!
- Maximum moves from 11 to 12 a gain of +1
On average the 1d12 roll only gain 0,99 damage yet we finally get the chance to roll <=4 below 5%! So one could say that this Savage Attacker feature justifies the use of a Great Axe for some extend. It helps you mitigate those low results, somewhat.
It would be interesting to see how both Great Weapon fighting style and Savage Attacker would combine statistically. Yet since the edge from savage attacker for 1d12 is rather low and the advantage from GW fs is in favor of 2d6, I would expect to 2d6 to be still ahead. (Let me know if you want this math done)
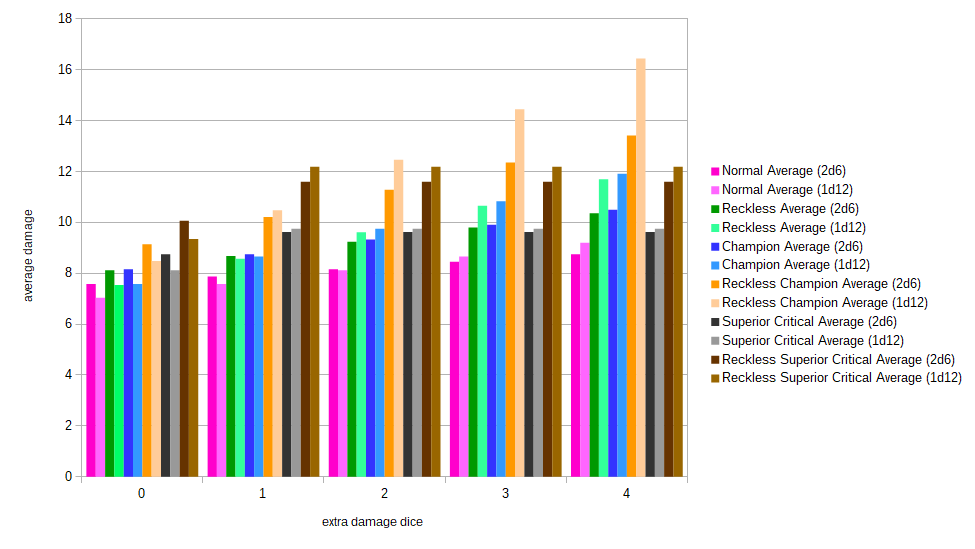
So the only thing left to look at is the critical hits
As pointed out in previous answers the 1d12 great axe only real redeeming qualities lie in the benefit of added critical hit dice and of and expanded critical hit range.
I'll first give the results and I'll include some graphs to show how small the differences are on average.
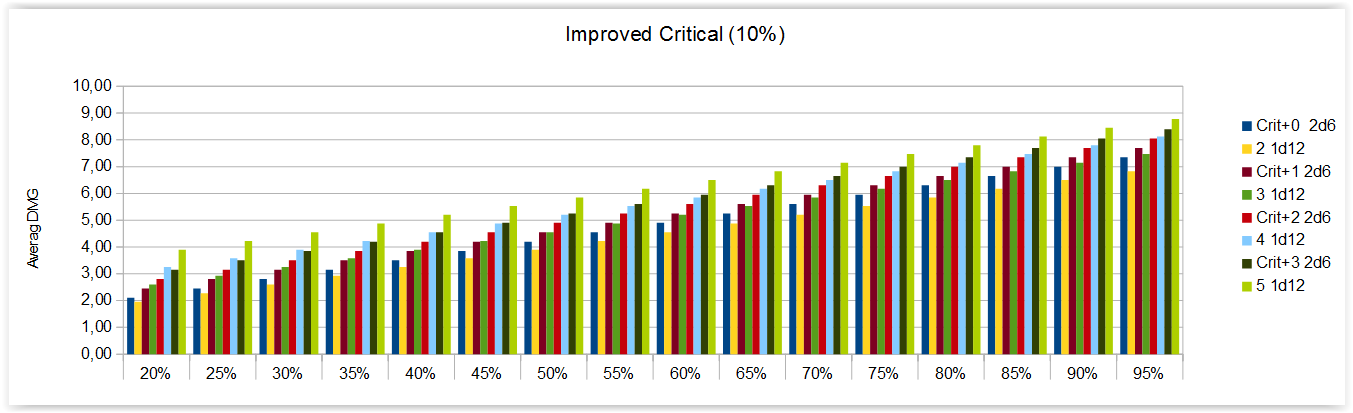
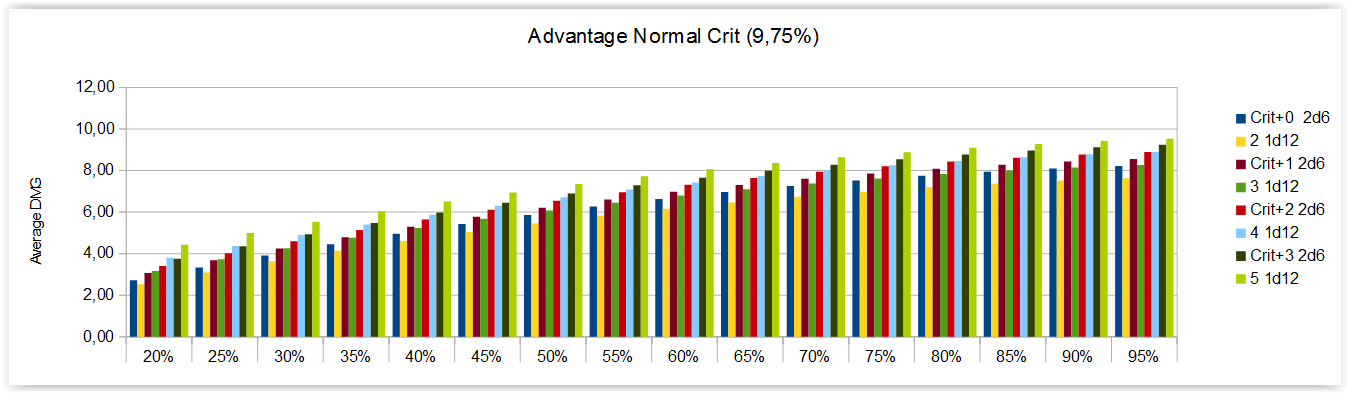
With normal critical range and no advantage you need to add three critical hit dice to have some advantage from using a great axe.
With either Improved Critical or Advantage on the attack roll, the great axe becomes beneficial from +2 critical dice.
If you combine Improved Critical and Advantage on the attack roll, the great axe becomes beneficial from +1 critical dice.
Yet one must understand that these are average results, which means that they rely on the damage spike of the critical hit to increase the average damage dealt. Yet all damage done beyond the HP left of your target, is wasted. So having a better potential for dealing more spike damage only is relevant when you have enemies with enough HP for it to count.
My personal experience has been so far that my Improved Savage Attacker Feature as allowed my character to one-hit mooks more reliably, which is really the benefit you are looking for: increasing your action economy in combat. Without any ways to reduce the chance of rolling low, a great axe isn't a reliable weapon if you want to take out enemies consistently.