As the variety of approaches here show, the rules for this aren't as clear as one might hope. Here's the understanding we have at our table.
First of all, the relevant rules from the Player's Handbook (p. 204) on the shape of a cone:
A cone extends in a direction you choose from its point of origin. A cone's width at a given point along its length is equal to that point's distance from the point of origin. A cone's area of effect specifies its maximum length.
A cone's point of origin is not included in the cone's area of effect, unless you decide otherwise.
One might expect that the rules from the Dungeon Master's Guide (p. 251) on translating areas to a grid might help:
Choose an intersection of squares of hexes as the point of origin or an area of effect, then follow its rules as normal. If an area of effect is circular and covers at least half a square, it affects that square.
But here we have our first bit of confusion: Is the area of effect of a cone circular? It is in 3-d space, but we usually only care about a 2-d grid projection. Honestly, even the bit about starting at an intersection seems weird to me, particularly for the spell Burning Hands that you're asking about that already states that "your outstretched fingertips" are the point of origin. So I'm mostly going to ignore the DMG rule, as I think it adds more confusion for this case than it solves.
Also, our group uses the "Optional Rule: Diagonals" (DMG p. 252), which alternates between counting 5 and 10 feet when counting diagonally. It makes the square root of 2 equal to 1½, which is certainly closer than the "core" grid rules give you (where it equals 1) and makes some of this math make a bit more sense.
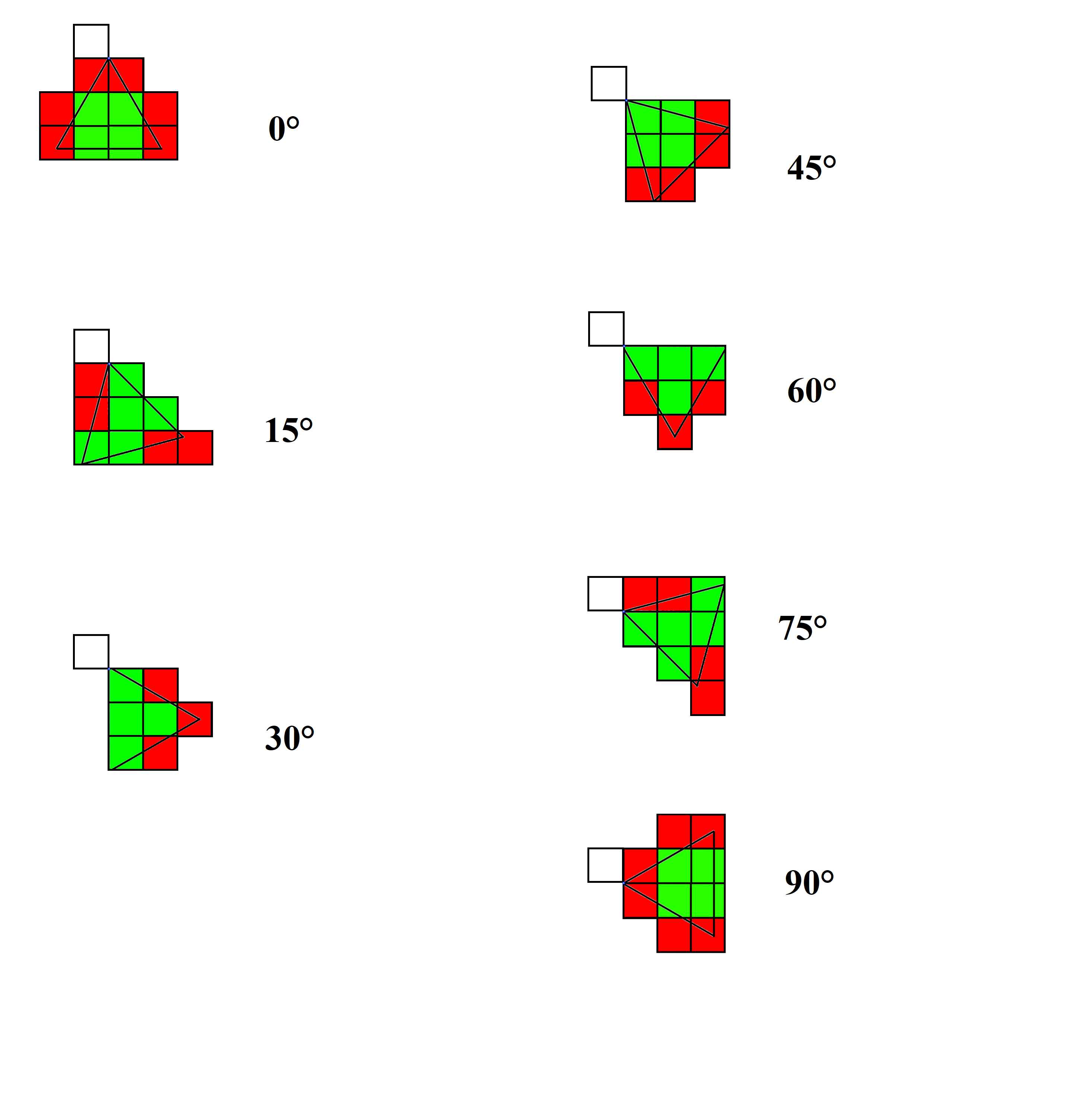
So, now that we've looked at the rules, how do we apply them? In particular, the definition that "a cone's width at a given point along its length is equal to that point's distance from the point of origin" means I expect that a 15′ cone effect would cover 6 squares of the grid: 1 space (5′) away from you it should be 1 square (5′) wide, 2 spaces (10′) away from you it should be 2 squares (10′) wide, and 3 spaces (15′) away from you it should be 3 squares (15′) wide. And you could, if you wanted to, add a seventh square of yourself, if you choose to include the point of origin in the cone's area of effect.
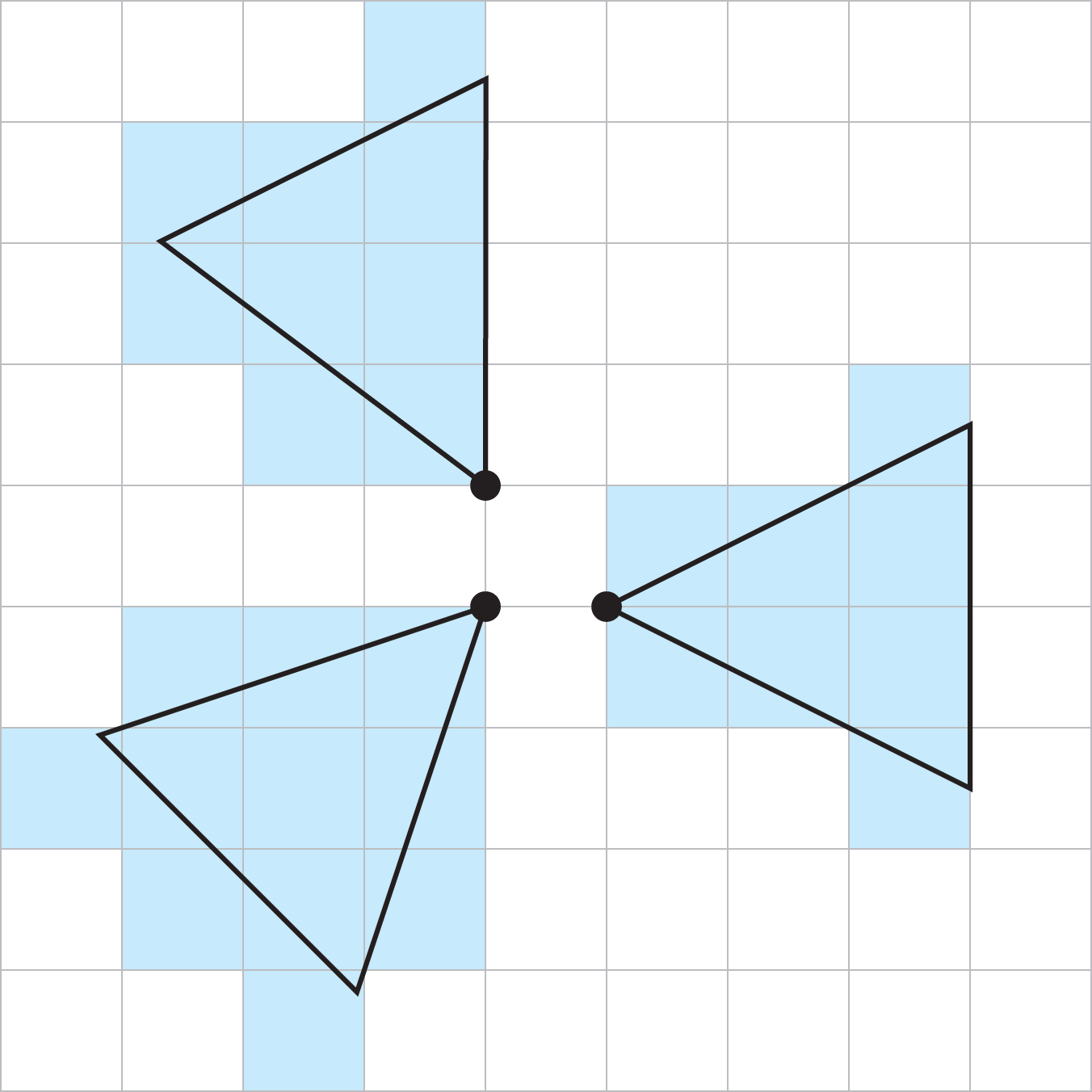
Since we're on a grid, I'm fine with limiting direction to one of the eight "main" directions, either adjacent or diagonal to you. Let's start with going in an adjacent direction:
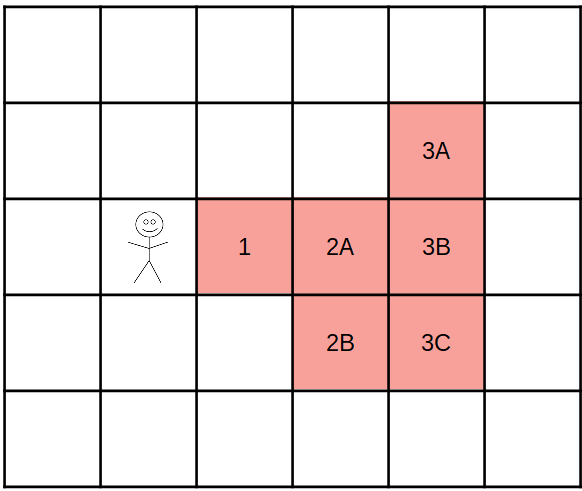
Of course, this can be mirrored (with box 2B north of 2A instead of south of it), or rotated in 90° increments to any of the 4 adjacent directions. Here's my logic for why this works:
- Box 1 is 5 feet away from the caster, and it's 5 feet wide.
- Boxes 2A and 2B are 10 feet away from the caster, and the effect is 10 feet wide at that point.
- Boxes 3A, 3B, and 3C are 15 feet away from the caster, and the effect is 15 feet wide at that point.
Certainly this is a bit of an "intuitive" approach, and not based on overlaying a triangle on the grid or anything, but I think it works with the way D&D does "grid math", with squares the correct distance away being affected.
If you want to "designate a corner" to try to apply the DMG rule, I think calling the effect emanating from the northwest or southwest corners of square 1 makes some amount of sense, but like I said I don't think doing so adds a lot of clarification.
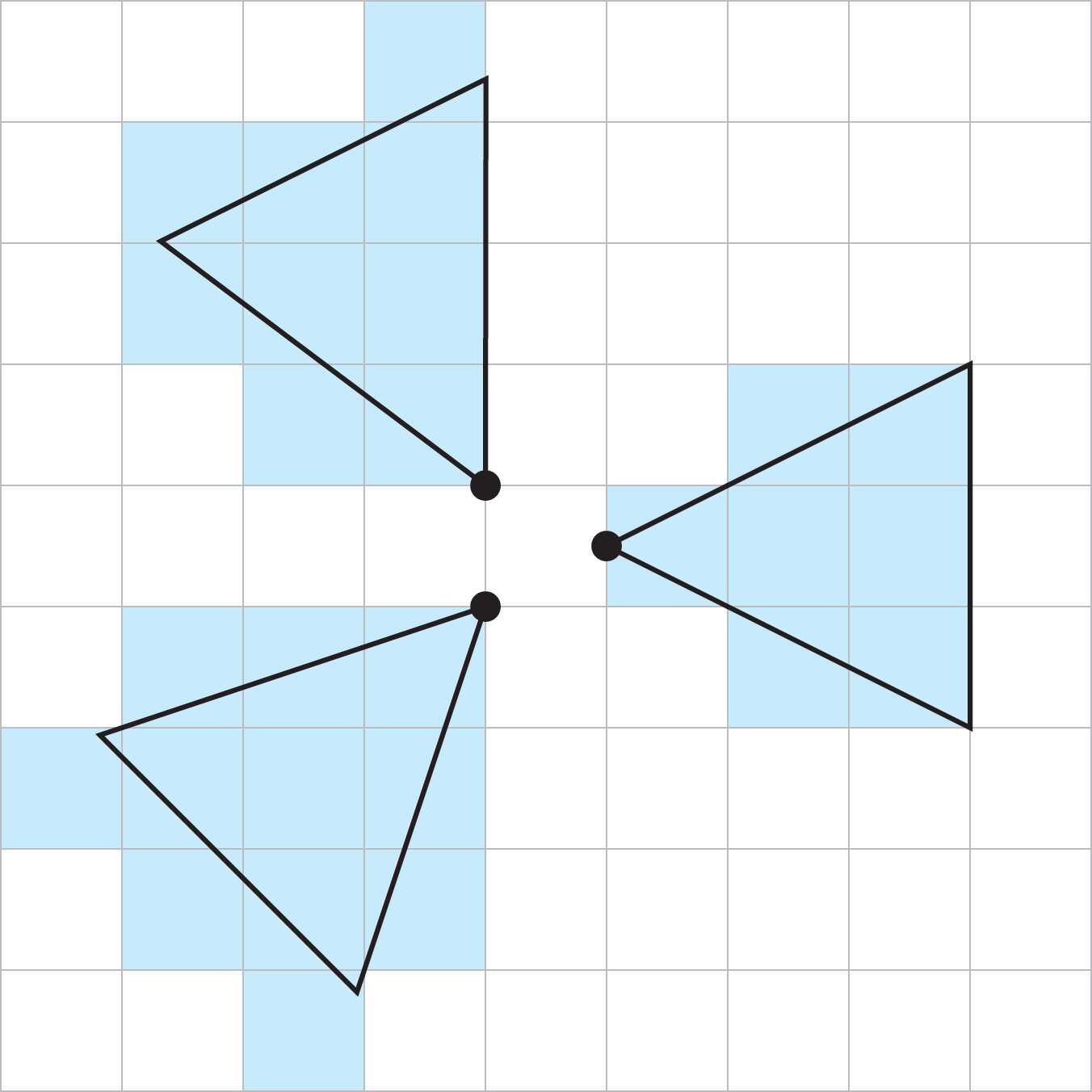
What if the caster wants to go diagonally? Here's how I see it working:
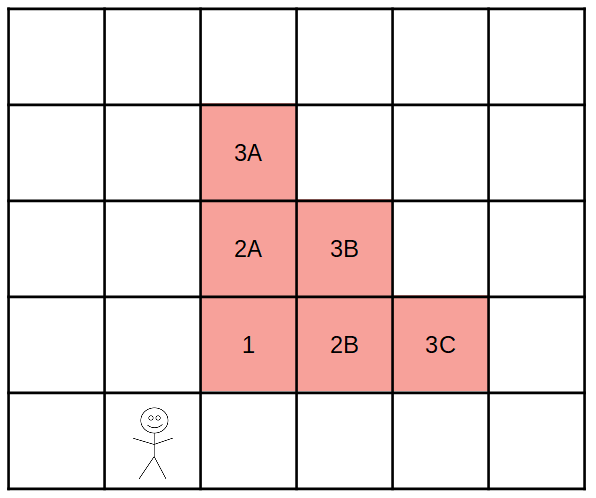
Again of course, rotate in 90° increments to go to a different diagonal.
- Box 1 is 5 feet away from the caster, counting diagonally, and as just itself it's 5 feet wide.
- Boxes 2A and 2B are 10 feet away from the caster, and as they're each 5 feet away from each other, and there's two squares, the cone is 10 feet wide at that point.
- Boxes 3A, 3B, and 3C are 15 feet away from the caster. (And this is why using the diagonal option is helpful, so that 3B is 15 feet away and not 10.) The cone could be considered a bit too wide here, as 3A is 15 feet away from 3C (meaning that the total "width" could be considered 20 feet, of the 5 feet of one square plus the 15 feet it takes to get to the other side). However, as it's three squares, each 15 feet away from the caster, I think it makes the most sense. And if you weren't using the Diagonals optional rule, then the width of the cone there would be 15 feet.
If your group wanted to have the caster omit one of squares 3A or 3C when casting diagonally, I could certainly see that making some sense, but my instinct is to just allow it so that there are three squares affected 15′ away.
At least diagonally it's clear which corner the effect emanates from for the DMG rule.
Again, this is more based on counting and intuition rather than geometry, but using a grid with D&D in general seems to be based more on counting squares than trying to measure like a tactical wargame. Working like this works well enough for our group.