Short answer
If you have advantage most of the time, Elven Accuracy is simply a superior choice for every AC everytime. Even if you don't, Elven Accuracy is still probably superior.
General math
Your average damage is given by $$D = P \cdot d + P_c \cdot d_c$$ where \$P\$ is the final probability of hitting and \$P_c\$ is the final probability of a critical hit, \$d\$ is the average damage on hit and \$d_c\$ is the average damage on critical. These values will change according to the build. Let's do the math to each one:
Elven Accuracy
Elven Accuracy states
Whenever you have advantage on an attack roll using Dexterity, Intelligence, Wisdom, or Charisma, you can reroll one of the dice once.
Per your question, I'll assume this triggers 100% of the time.
Let \$p\$ be the probability of a single dice beating the enemies' AC with your Attack hit modifier, given by \$p = (21 - (\textrm{AC} - B)) \cdot 5/100\$1, where \$B\$ is your hit bonus. You also have a probability of critting \$p_c = 5\%\$.
With triple advantage, you will crit with probability $$P_c = 1 - (1 - p_c)^3$$ and hit with probability $$P = 1 - (1 - p)^3 - P_c.$$ This essentially means you will hit normally whenever you don't miss and you don't crit.
Your average damage with 1d8 + 1d6 is 8. The bonus damage will depend on using sharpshooter or not, as well as \$B\$.
I could do the math by hand, but why would I? In the end there is a plot of everything together in funciton of AC.
ASI
This essentially changes the values of \$d\$, \$d_c\$ and \$B\$ by a +1 modifier. Without triple advantage, you still have advantage, which leads us to $$P = 1 - (1 - p)^2 - P_c$$ and $$P_c = 1 - (1 - p_c)^2.$$
Results
First, let's check your current state. With \$B = 7\$ (or \$8\$ if you get ASI) and average damage with +5 (or +6, or +15, or +16, depending on whether you get ASI and use SS or not), this is the graph. X axis is the AC, Y axis is the average damage.

EA is superior above 8 AC (which is essentially against every monster in the game) in the conditions you mentioned (with 100% advantage). At ACs higher than 20, you should stop using Sharpshooter and hit normally.
What about the +2 weapon?
That will change your modifiers by +2. Let's check how it changes your things.
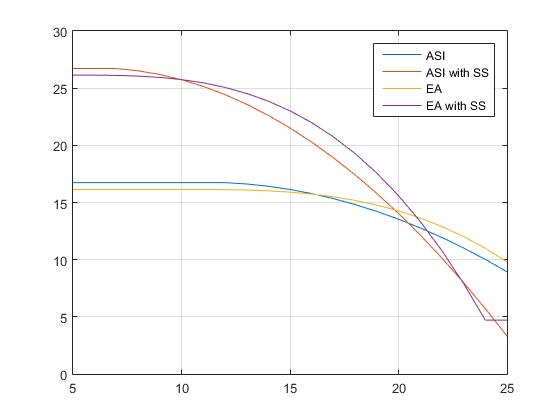
EA is still the best option. For ACs lower than 10 (which are usually zombies or other easy to hit monsters) you get a really low bump on the average, while at the most common range of ACs (12-18) EA is superior. For low ACs you should be using SS and, again, later you just stop using it. This time, if the AC is higher than 21, not 20, though.
Note that the easier it is to hit (for example, check the curves for low AC without SS), the worse the triple advantage gets. If you didn't get SS, EA could actually be a bad choice, but the combo of highly increasing the damage while decreasing the to hit and then highly increasing the to hit is really strong.
What if you don't have advantage?
Obviously then EA will be worse, since it provides no bonus whatsover. How bad is it though? Is it so bad that if you don't have advantage it sucks?
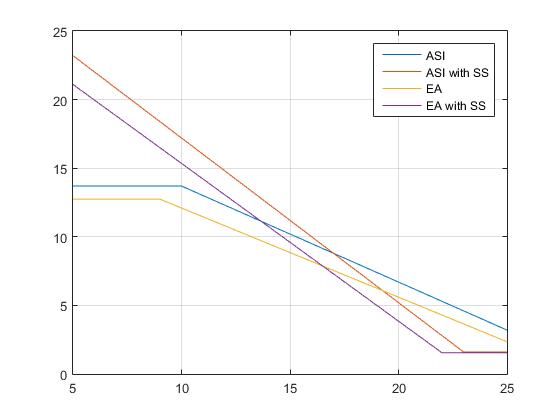
With SS, it provides a -2 average damage. Without it, it's a -1 average damage. With advantage, the bonus you get is close to it, slightly superior in common AC ranges. As a conclusion: if you are able to get advantage in more than 50% of your attacks, EA is certainly superior. Even if you don't, remember that EA gives you +1 ASI either way.
Note: this average damage is for a single attack. Sneak Attack only procs in the first attack, though, so the extra attack it changes the damage a little. I've rerun it and it's not meaningful in the results. EA is superior, period.
1 This should be clipped at 0.05 and 0.95, since you always have the 1 = miss and 20 = hit.



