Is there an accepted likelihood of hitting that is consistent across tiers, and how has it been determined?

Question

One can easily calculate DPR given a specific build (knowing proficiency bonus, ability scores, other relevant factors) and the AC of opponents, leading to spreadsheets with a matrix of customizable parameters. But what if one wants to calculate an average DPR considering typical build choices against typical CR encounters? As character attack bonuses go up, so do opponent ACs, so is there commonly used rule of thumb for an average chance to hit?

For example, the following sources use chances of hitting that are apparently intended to be applied across tiers:

• 50%: What is the expected DPR of an animate object spell cast on daggers? ("11+ on the d20 is required to hit")
• 60%: https://www.youtube.com/watch?v=zg0bAl1WPGQ&t=9m5s ("This warlock's going to have about 60% chance to hit. And, honestly, that ends up being pretty close to the average.")
• 65%: https://www.enworld.org/threads/guide-my-word-is-my-sword-the-paladin-guide.474035/ (used several places throughout the guide)
• 65%: Weapon attacks compared with damaging cantrips? based on:
  • ASI goes into attack ability
  • Attacks will be made against average AC of a CR = character level, as seen on DMG274

Is there a widely agreed upon likelihood of hitting in a generic, CR-appropriate encounter (e.g. 50%, 65%, etc.)? Is there one that is consistent across levels or tiers? How is this value arrived at? (E.g. likely character attack bonuses, AC of CR-appropriate opponents…)

Answer 1

Disclaimers:

1. I've never seen an "accepted" or "general" number thrown around, so this answer represents both my own feelings, and my own inference given the available data.
2. All monsters are different, so we can only work in averages based on given guidelines.
3. "CR-appropriate" encounters comprised of multiple creatures will pull your hitting average up by 5% when crossing the following CR lines: 3-4, 4-5, 7-8, 9-10, 12-13, and 16-17; fighting creatures above your party CR will have the opposite effect.

Players should hit about 65% of the time

Using the DMG guidelines explained in this answer for Average AC at a given CR and a combat-optimized stat-array human, starting at 16 in their attack stat...

CR 0-3 : +5 to hit vs 13 AC requires an 8 or better; 65% to hit.
CR 4 : (ASI) +6 to hit vs 14 AC requires an 8 or better; 65% to hit.
CR 5-7 : (Proficiency +3) +7 to hit vs 15 AC requires an 8 or better; 65% to hit.
CR 8 : (ASI to 20) +8 to hit vs 16 AC requires an 8 or better; 65% to hit.
CR 9 : (Proficiency +4) +9 to hit vs 16 AC requires a 7 or better; 70% to hit.
CR 10-12 : +9 to hit vs 17 AC requires an 8 or better; 65% to hit.
CR 13-16 : (Proficiency +5) +10 to hit vs 18 AC requires an 8 or better; 65% to hit.
CR 17+ : (Proficiency +6) +11 to hit vs 19 AC requires an 8 or better; 65% to hit.

You can get ahead of the curve slightly by using either rolled stats or being a fighter, but you're still going to max out your attack ability and then you're back on track at level/CR 8.

And starting with a 14 (or 15) in your attack ability only sets you back to 60% until your ASIs catch up, at which point, you're back to 65%.

This jibes with what limited amount that I understand about game-building. The players should feel like they're effective more often than not, but not so much as to guarantee success.

Magic items can move you forward a bit

If you use the following table as a guideline from the DMG (p 135), you're going to move ahead five percentage points for every "+1" you gain to your attack.

MAGIC ITEM RARITY

Rarity	Character Level
Common	1st or higher
Uncommon	1st or higher
Rare	5th or higher
Very Rare	11th or higher
Legendary	17th or higher

Magic items are entirely optional and furthermore doling them out is DM dependent, so I'm not going to factor them into the chart above. Although, if you can get your hands on a +3 weapon at level 9, you're going to average a whopping 85% to hit!

Answer 2

Average to-hit probabilities by tier and level

This calculation uses all available monsters from the Monster Manual as a baseline for monster ACs in the absence of game world or campaign specific context.¹

• Tier One (Level 1-4): 64%²
• Tier Two (Level 5-10): 70%
• Tier Three (Level 11-16): 75%
• Tier Four (Level 17-20): 79%

Over tiers, the likelihood to hit improves, mostly due to access to better magic items³. If you calculate without magic items, then the result would closely match the 65% proposed in the accepted answer across all tiers.

Most campaigns are playing in tier one and two, and the average for the first ten levels of play is 66%. Across all tiers of play, the weighted average to hit probabilty is 68%. If you want a single number rounded to multiples of 5% for character to-hit probability in 5e, you could use 70% as a rule of thumb.

How is this calculated?

These calculations are for the core rules.

Tier probabilities above were calculated by averaging the to-hit probabilities for all levels within the tier, weighted by the number of monsters for each level.

Average AC: The average AC for each challenge rating is calculated by averaging the ACs from all monsters in the Monster Manual that have that challenge rating. (Additional, optional monsters from sources like Mordenkainen's tome of foes are not included). Because the AC is an averaged number, it is often not a round number. Obviously, in no individual fight would you encounter a monster with such an AC, but we look at averages.⁴

PC to hit: The to hit rate assumes that characters are built using either the standard roster or point buy to put a 15 on the ability that is used to determine to hit and a race that optimizes the ability by adding one or two points to the ability, for a total of +3 ability bonus. It assumes that this ability is maximized in level 4 and 8 with ability score increases. It further assumes that in level 5-10, the character has access to a +1 magic weapon, in level 11-16 to a +2 magic weapon, and from level 17 on to a +3 magic weapon, based on the rarity of such weapons and on the recommendations for starting equipment magic items on p. 38 DMG.

Monsters is the number of monsters with that CR

Delta is the numerical difference between AC and PC to hit.

Chance to hit is 55% (the probability to hit a given AC with a to-hit number that is numerically identical) plus the Delta times 5%.

A key question for this is what monsters to assume as encounters for a given level. This table assumes the average monster encountered to be of the same CR as the level of the characters. Many encounters consist of multiple monsters of lower CR, or in some cases dangerous encounters can be with monsters of a higher CR than the average party level. In the absence of statistics for how common those situations are, this avoids arbitrary assignment of likelyhoods. For this reason, monsters with a CR greater than 20 were not considered in the calculation (although they are listed on the table).¹

CR	Avg AC	Monsters	PC to hit	Delta	Chance to hit
0	11.0	30	15	4.0	75%
1/8	12.4	23	15	2.6	68%
1/4	12.0	38	15	3.0	70%
1/2	12.3	33	15	2.7	68%
1	13.2	31	15	1.8	64%
2	13.1	51	15	1.9	65%
3	14.4	26	15	0.6	58%
4	13.4	16	16	2.6	68%
5	15.0	30	18	3.0	70%
6	15.4	14	18	2.6	68%
7	16.1	9	18	1.9	64%
8	15.6	14	19	3.4	72%
9	16.7	10	20	3.3	72%
10	17.3	8	20	2.8	69%
11	16.9	8	21	4.1	76%
12	15.7	3	21	5.3	82%
13	17.4	9	22	4.6	78%
14	18.5	4	22	3.5	73%
15	18.3	4	22	3.8	74%
16	19.0	5	22	3.0	70%
17	19.0	7	24	5.0	80%
18	20.0	1	24	4.0	75%
19	19.0	1	24	5.0	80%
20	19.7	3	24	4.3	77%
21	20.3	4	24	3.8	74%
22	21.5	2	24	2.5	68%
23	21.0	4	24	3.0	70%
24	22.0	2	24	2.0	65%
30	25.0	1	24	-1.0	50%

---

¹ Depending on the game world, certain kinds of monsters may be more common than others. In most campaigns, the ACs of goblins, skeletons, ogres and guards will likely feature in more encounters than the ACs of octopi, tridrones, and intellect devourers. It might be possible to calculate this more exactly by using the actual encounters from published campaigns to determine the average CRs at each expected character level for that specific campaign, this was not done above.

Empirical Encounter AC data

Well actually, I did it for one example: below is the distribution of monsters encountered over the whole of the Tomb of Annihilation campaign module that goes from level 1 to 11, 485 encounters in total. This is not perfect, as it just tallies all of the fixed encounters in the module, plus counts each random encounter once, and not all of the random encounters are exactly equally probable. But given the frequency of encounter checks, they are all improbable enough that you would not expect to have each more than once on average, so it should be a reasonable approximation. The "Sum of #" bars show how many monsters of that CR can be encountered (blue numbers), the red "Average AC" line shows the average AC for these monsters (red numbers).

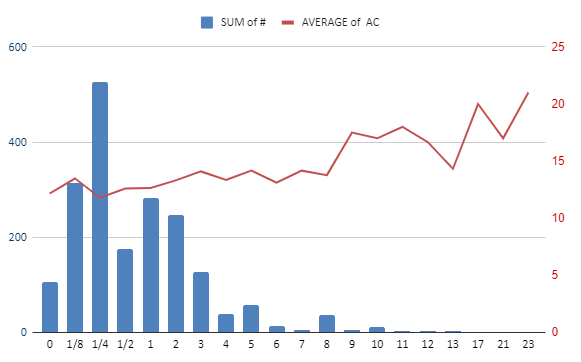
What you can see is that the average AC of the monsters encountered is relatively flat and slowly increasing matching the theoretical values predicted above quite well. Encountering a monster a couple CRs higher and lower will not make a huge difference. You also can see that the vast majority (about 90%) of creatures encountered in this campaign is of CR 3 or lower, because these often tend to appear in somewhat larger groups. Above CR 12, we are dealing with unique monsters encountered as singletons.

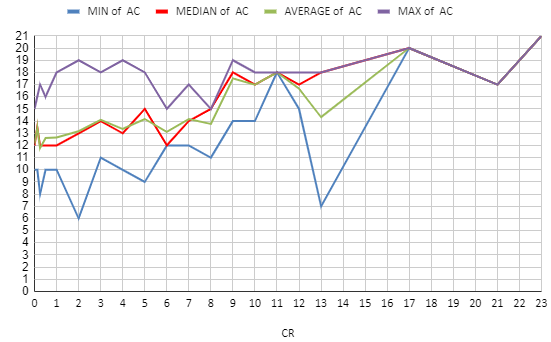
The chart below shows the span of ACs encountered at each challenge rating. The lowest ACs are 6 (a couple of Yellow Musk Creepers at CR 2) and 8 (a lot of Zombies in the jungle at CR 1/4). The higest ACs are 19 for a few Will-o'-the-Wisps (likewise at CR 2, the widest spread) and a couple of unique monsters like a dragon turtle, a lich or Acererak. The outlier that is dragging the average down at CR 13 with only 7 AC is the Atropal. This of course is expected: not every monster will match the average AC, individual encounters can have much higher, or much lower numbers.

What is evident is that the median (that is, the most often encountered AC) is quite close to the mean across the entire range up to where we get to deal with unique encounters after CR 12 (demons and beholders all with AC 18 vs the Atropal with 7). That is, for most fights, the average AC number per CR is a pretty good approximation to the commonly encountered AC. The outliers are rare.

(In case you are curious what the most common monsters in this module were, the top 10 are, in order: Guard, Grung, Gargoyle (dozens of them line the rim of a certain cliff), Aarakocra, Yuan-ti Pureblood, Bandit, Yuan-ti Malison, Animated Armor (there is a whole room full of them in a crypt), Tribal Warrior, and Zombie.)

² Tier One calculated based on CR 1-4 opponents. Increases to 67% if calculating based on CR 0-5 opponents.

³ As this survey of over 750 respondents shows, magic items are the norm in D&D campaigns. Over 70% reported that magic items are very common or common and frequently found as rewards from quests or as treasure, and over 95% respondents reported them being given out at least at significant milestones in the campaign. Under 1% of the population reported playing without magic items. In addition, over three quarters of respondents supported magic item shops.

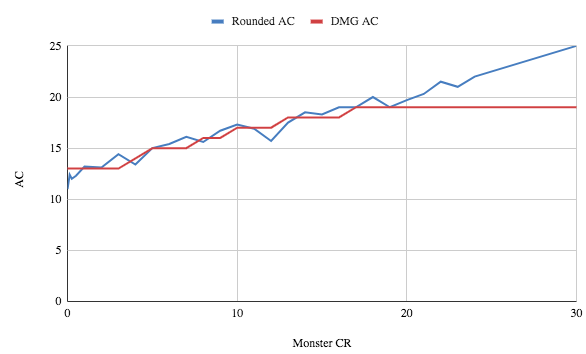
⁴ Another approach is to look at the monster building guidelines in DMG p. 275 to determine what AC per CR "should be", but that is not used here. Below is a comparison, which shows that both are relatively similar, as long as you stay away from very high CRs (rounded AC is to one digit after the decimal point as above for Avg AC):

Answer 3

Your question is inherently flawed and unanswerable

Let's start by stating that there is no real "typical build" for any of the classes, because there's no way to say what is and isn't 'typical':

I could make a level 4 fighter with 18 dex and archery fighting style to gain +8 to hit, or I could play a sword&shield tank fighter with only 15 strength and have half that chance to hit bonus, yet neither of these builds would be considered atypical.

The real problem is that there's also no generic AC to hit against. An Ogre is CR2 and only has an AC of 11, while an Azer is also a CR2 creature and that one has an AC of 17. Challenge ratings take both defensive and offensive features into their calculation, so there is no real way to truly say what AC a specific CR creature has, it depends on how their stats are distributed.

The DMG list on page 274 is not, in any way, useful for this purpose. Other answers in the past may have suggested using that list, but that is not how that list works. A creature's final CR depends on its AC, HP and expected damage output, plus an adjustment based on playtesting. If you flip through the monster manual, you'll find that the vast majority of monsters do not fit their suggested AC from the DMG list, because they have more or less HP, or deal more or less damage. It might have immunity to non-magical weapons, it might have resistances, there are a lot of things that go into CR that in no way tie to AC.

Do we go by the +8 archer hitting an Ogre? Do we go by the sword and shield fighter trying to hit an Azer? That's a massive difference in chance to hit, even though both are level 4 characters trying to hit a CR2 creature.

People who throw around a "has a 65% chance to hit a challenge appropriate level encounter" facts are mostly just pulling them out of thin air, because encounters are rarely just one static enemy of an appropriate CR. Ten goblins is often more likely as an encounter than one creature by itself.